|
|
|
WELCOME TO THE PHARMACY WORLD
Introduction to the UV/Visible Spectrophotometer
Optical System Diagram
The UV-Visible spectrophotometer uses two light sources, a deuterium (D2) lamp for ultraviolet light and a tungsten (W) lamp for visible light. After bouncing off a mirror (mirror 1), the light beam passes through a slit and hits a diffraction grating. The grating can be rotated allowing for a specific wavelength to be selected. At any specific orientation of the grating, only monochromatic (single wavelength) successfully passes through a slit. A filter is used to remove unwanted higher orders of diffraction. (Recall the experiment you did last semester on Atomic Spectra) The light beam hits a second mirror before it gets split by a half mirror (half of the light is reflected, the other half passes through). One of the beams is allowed to pass through a reference cuvette (which contains the solvent only), the other passes through the sample cuvette. The intensities of the light beams are then measured at the end.
Beer-Lambert Law
The change in intensity of light (dI) after passing through a sample should be proportional to the following:
(a) path length (b), the longer the path, more photons should be absorbed
(b) concentration (c) of sample, more molecules absorbing means more photons absorbed
(c) intensity of the incident light (I), more photons mean more opportunity for a molecule to see a photon
Thus,
dI is proportional to bcI or
dI/I = -kbc (where k is a proportionality constant, the negative sign is shown because this is a decrease in intensity of the light, this makes b, c and I always positive.
Integration of the above equation leads to Beer-Lambert's Law:
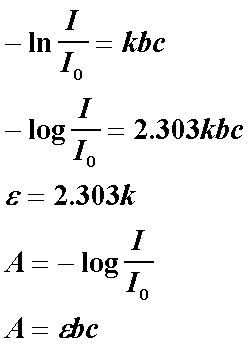
A is defined as absorbance and it is found to be directly proportional to the path length, b, and the concentration of the sample, c. The extinction coefficient is characteristic of the substance under study and of course, is a function of the wavelength. Molecules strongly absorb only in some regions of the electromagnetic spectrum. The photon carries a specific amount of energy defined by its wavelength (Recall Planck's equation: E = hc/wavelength). The molecule will only absorb a photon if the energy it carries matches a certain amount the molecule can use. In the ultraviolet-visible region, this energy corresponds to electronic excitations (promotion of electrons from occupied orbitals to unoccupied orbitals). The longest wavelength (the least energy) therefore corresponds to the energy difference between the ground and the first excited state (or promotion of an electron from the highest filled orbital (HFO) to the lowest unfilled orbital (LUO)). Let us take the example of a nitrogen (N2) molecule. The highest filled orbitals is the sigma bonding orbital while the lowest unfilled orbitals are the degenerate antibonding pi orbitals. Thus, a photon of energy which corresponds to the difference in energy between the bonding sigma(2p) orbital and the antibonding pi(2p) orbitals can cause a sigma --> pi* transition.
Let us look at this situation much closer: In the ground state, N2 has a bond order of 3 (triple bond) but as soon as a photon of the right wavelength is absorbed, one of the electrons can get promoted to one of the pi* orbitals, thus, reducing the bond order to 2. This excitation is not dissociative. Compare this to the case of a hydrogen molecule. The ground state of H2 is described by having two electrons in the bonding sigma(1s) orbital. This orbital is likewise the highest filled orbital. The lowest unfilled orbital is the antibonding sigma*(1s) orbital. Thus, electronic excitation by a photon of energy equivalent to the difference in energy between the sigma(1s) and the sigma*(1s) leads to an excited state where there is one electron in the sigma(1s) and the other electron in the antibonding sigma*(1s) orbital. Excitation in this case therefore leads to a molecule in which the bond order is zero. The molecule breaks up.